At the same time, many audio-visual enthusiasts and enthusiasts also hope to understand such content. Therefore, "New Life of Audio-visual" has set up a special topic to introduce the basics of sound science comprehensively and help people to understand acoustics and sound technology more conveniently.
As long as you are not a deaf person, you will have a specific concept of sound. But for most people, the physical characteristics of sound are unfamiliar. Here is a brief explanation of how sound is generated and transmitted.
振动 Vibration and frequency objects Under the influence of external forces, the reciprocating motion along a straight line or curve and passing through its equilibrium position is called vibration. The vibrating body vibrates back and forth once and is called a full vibration process. The total number of vibrations that the vibrating body completes in 毎 seconds is called frequency. The commonly used unit is called Hertz, which is called Hertz (Hz). 10 times / sec is l0Hz, 1000 times / sec is 1KHz. The frequency is determined by the speed of the vibration. The more vibrations per second, the higher the frequency and vice versa. The term "high frequency sound" and "low frequency sound" that we are used to is a relative concept. The high frequency is about 1 kHz or higher, and the low frequency is about 440 Hz or less.
振动 The vibration of sound waves and wave velocity objects will propagate in the medium (such as solids, gases, liquids) at a certain speed. This process of propagation is called sound wave diffusion. The speed of sound wave propagation varies with the density of the medium. For example, the speed of sound in air at 15 ° C is 344 m (meters) per second, l437 m per second in water, and 3013 m per second in the skull.
振幅 The maximum value when the amplitude and attenuation of the vibrating object deviate from the equilibrium position during vibration is called amplitude. The magnitude of the amplitude is determined by the external force that causes the object to vibrate. Within the elastic limit, the larger the external force, the larger the amplitude. During the vibration process, the object loses energy due to the resistance caused by overcoming the friction and its own elasticity, and the amplitude gradually decreases with time until it disappears. This is the attenuation phenomenon of vibration. The vibration of most substances is attenuated, and the vibration can only continue if the energy is replenished. The size of the sound is related to the magnitude of the amplitude.
振动 Vibration curves, sine waves, and wavelengths are graphically described to describe images of vibration or wave states, called vibration curves or wave curves. In modern conditions, this curve is usually obtained using an instrument.
If the vibration curve changes according to the law of the sine of the trigonometric function with time, it is called a simple harmonic vibration, and its waveform is a sine wave. The sine wave is the most basic type of vibration in all vibrations. This type of vibration is caused by the vibration generated when the tuning fork is lightly tapped.
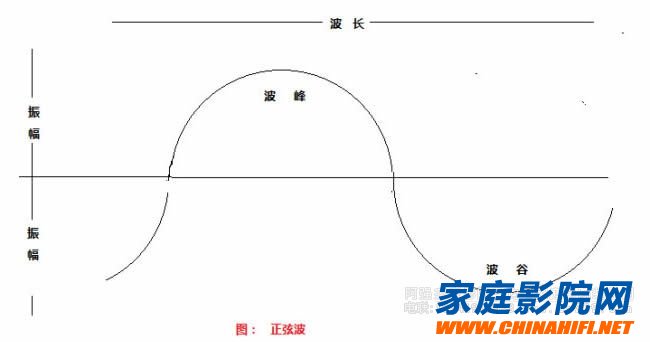The highest point of the sine wave bulge is called the peak; the lowest point of the recess is called the trough. In the figure, the horizontal axis represents the direction of propagation from left to right, and the distance between the peak or trough to the horizontal axis represents the amplitude. On the horizontal axis, from the equilibrium position, each time the wave passes through the valley, it returns to the equilibrium position, representing the wavelength. Sound waves of different frequencies have different wavelengths. The higher the frequency, the shorter the wavelength, and the calculation method of the same wavelength: V/f=h, where: f=frequency, V=wave speed 344m/s, h=wavelength, for example 200Hz The wavelength is 1.72m. Different wavelengths have a great influence on the position of the human ear to distinguish the sound.
ä½ç§» Displacement, phase, and phase difference <br> The movement of the vibrating body during vibration or the position of the medium in the process of sound wave propagation is called displacement. An amount used to indicate the state of displacement of a vibration (fluctuation) at a certain time is called a phase. This amount is usually expressed in terms of angle, called phase angle, referred to as phase.
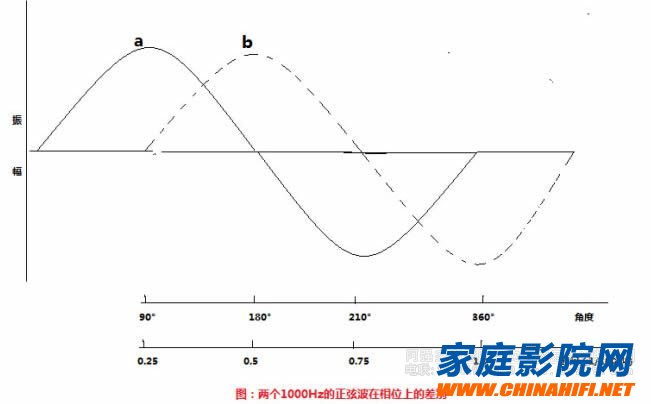
When the vibrating body is in the equilibrium position (ie, the position when no vibration occurs), the phase angle is generally 0. If it is a simple harmonic vibration, when the displacement reaches the maximum value, the phase angle is 90° or 270°, and the phase is the same. The same phase; the opposite phase is called the inversion point. For example, two tuning forks with the same frequency, if they are simultaneously hit, will produce completely consistent vibrations, and their acoustic phase will be exactly the same. If the time of the stroke is sequential, the sound waves generated by the two tuning forks will have a phase difference. The figure above is a sine wave of two l000HZ. The two sine waves differ in time by 1/4 cycle. When the b wave starts, the amplitude of the a wave has reached the maximum value. When the b wave amplitude reaches the maximum value, the a wave goes to the equilibrium position. Therefore, the phase difference between the two waves is 90°.
The phase difference of sound waves has an important influence on the sense of loudness and orientation of the sense of hearing.
▌ Forced vibration and resonance An object vibrates after being subjected to an external force. After the external force is suddenly eliminated, the vibration continues due to the elastic action inside the object itself. This vibration is called free vibration, and the frequency of free vibration is called the natural frequency. The free vibration of the A object causes the B object to vibrate according to the frequency of A. At this time, the vibration of B is called forced vibration, or forced vibration. For example, after the string is plucked on the violin, the string itself generates free vibration according to its natural frequency. When the free vibration of the string is transmitted to the resonance box of the violin through the bridge, the resonance box vibrates according to the frequency of the string. At this time, the resonance box The vibration is forced to vibrate.
Experiments have shown that if the frequency of free vibration is consistent or very close to the natural frequency of the forced vibrating body, the amplitude (volume) will quickly reach its maximum possible value. This phenomenon is called resonance, also known as resonance, which is called resonance in acoustics. Its sound is called a resonance sound. Of course, we also note that if the frequency of the free vibration is very different from the natural frequency of the forced vibrating body, the amplitude is reduced or even lost.
è€¦åˆ Coupling coupling is another property of resonance. When two or more vibration systems are combined with each other by resonance, a new vibration system is generated. This physical phenomenon is called coupling, and coupling is the sounding principle of many wind instruments. For example, the oboe relies on the vibration of the whistle to make a sound, but when the whistle is blown, not only the frequency is inaccurate, but also the tone is not beautiful, but when the whistle is connected with the body of the instrument, the tone is sounded immediately. This is because the whistle (spring vibration) is coupled with the vibration of the resonance tube (air column). The combined sound effect is also a type of coupling. Under certain conditions, the superposition of two synthetic waves of different frequencies (such as a musical instrument ensemble) will result in a third different frequency of sound. In addition, the generation of natural percussion is also related to the coupling phenomenon.
In the process of listening, the human ear will also have a coupling phenomenon, which will have an impact on the physiology and psychology.
When the two or more waves of the chopping are overlapped, they are called superposition, and the superposition is followed by the formation of a new wave called a composite wave. The superposition of multiple waves of different frequencies, different phases, and different amplitudes can produce a very complex synthesis phenomenon.
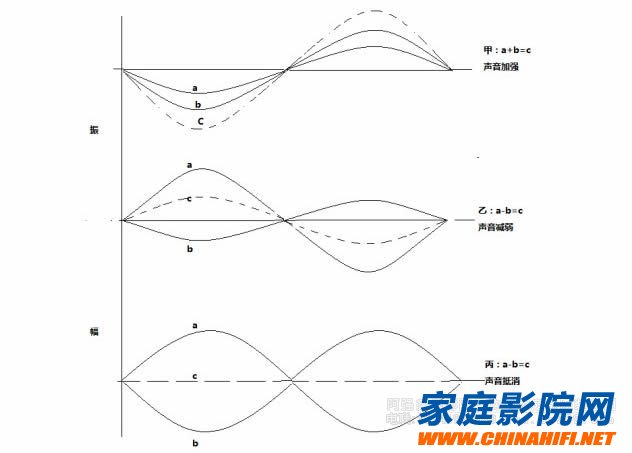The three simplest synthetic wave phenomena are listed. As shown in the above figure, the wave before the superposition is indicated by a solid line, and the synthesized wave is represented by a dotted line. In the figure, A: a wave and b amplitude are different, the phase is the same, and the sound is strengthened after the superposition. B: The amplitudes of the a and b waves are different, the phases are opposite, and the sound is weakened after the superposition. C: The amplitude of the a wave and the b wave are opposite in phase, and the sound is cancelled after the superposition. The superposition of waves plays a key role in the sensation of tone, volume and combined sound.
æ‹ Beat If the frequencies of the two waves are different but close, the amplitude of the synthesized will change periodically, called the beat. The sound of the beat that appears after the sound wave is synthesized is called the beat sound. Figure 13-4 shows the two waves of A and B and the synthesized waveform. The number of periodic changes in amplitude 毎 seconds after A+B synthesis in the figure is called the beat frequency, which is equal to the difference between the two wave frequencies.
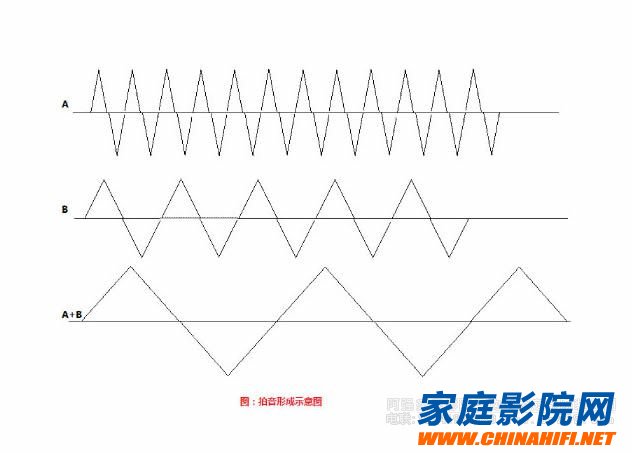
As shown in the figure, if the A wave is 50 Hz, the B wave is 40 Hz, 50-40 after synthesis is 10 (Hz), and the beat frequency is 10 Hz/s. The beat frequency is a phenomenon in which two waves interfere with each other. The beat phenomenon is often used to tune an instrument (such as a piano): When the two audio frequencies of the instrument are slightly different, a beat is heard, and when the beat disappears, the pitch is unified. In addition, some instruments (such as harmonica, accordion, organ, etc.) intentionally create a beat phenomenon, so that the sound undulations to achieve the purpose of beautifying the sound.
Summary: Mastering the basics of sound communication can better help us understand the acoustic characteristics of audio products and indoor buildings, and help to create an excellent audio-visual environment.
Fiber Optic Equipment,Fiber Optic Cable Drum,Fiber Optic Cable Reels,Fiber Optic Retractable Cable Reel
ShenZhen JunJin Technology Co.,Ltd , https://www.jjtcl.com